Scientific notation is a convenient way to communicate numbers that are very large or very small, which means it is important to know scientific notation in chemistry. Consider Avogadro’s number, the number of carbon atoms in exactly 12 grams of Carbon-12. We’ll learn more about Avogadro later, but his number happens to be 602,000,000,000,000,000,000,000.
That’s six hundred and two sextillion atoms! To say that’s a lot of atoms would be a huge understatement. If you stacked that many pennies, they would stretch to the sun and back over 3 billion times. Big numbers like this require the use of scientific notation.

Scientific notation begins with the first non-zero digit, followed by a decimal, followed by all remaining significant figures, followed by the appropriate power of 10.
How many significant figures are there in this number?
Write down the significant figures (there are 3 – but you already knew that!).
602
Put a decimal after the first digit.
6.02
Now, count the number of places you’d have to move that decimal to get back to the original number. In this case, that would be 23 places to the right. That becomes the power of ten that is multiplied by the first digits.
6.02 x 1023
That’s it!
Be aware that your calculator may have the ability to report numbers using scientific notation. However, as we have already learned, it doesn’t necessarily know which numbers are significant.
Be careful that you are only reporting the significant figures when using scientific notation.
Scientific notation can also be used for very small numbers.
For example, these are sodium chloride crystals magnified under a microscope. Notice that even at this high magnification, we still can’t see any individual atoms.
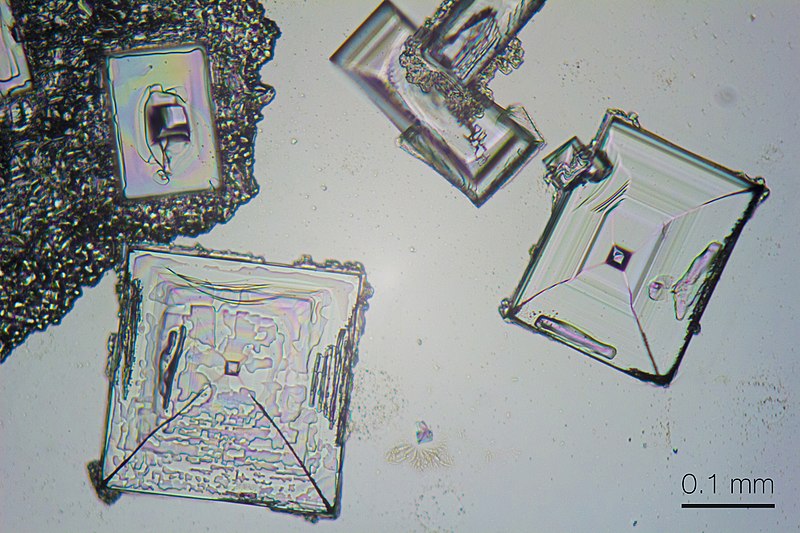
The graphic below shows the crystal lattice of Sodium Chloride. The radius of just one of the chloride ions in the lattice is 0.000000000167 meters. They are miniscule!
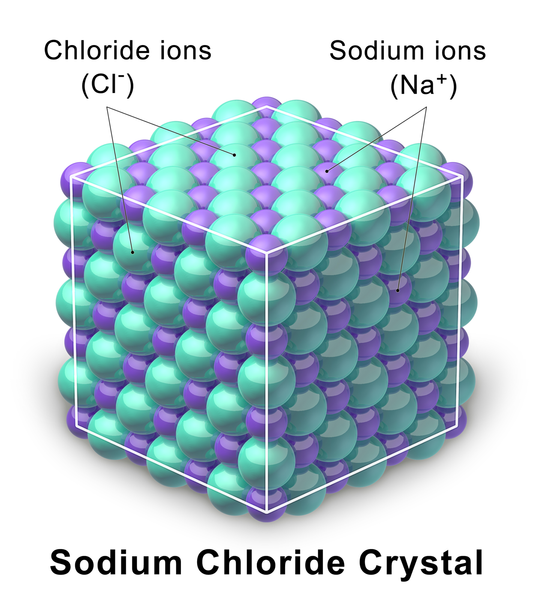
Let’s write 0.000000000167 meters in scientific notation.
First, write only the significant figures.
167
Put a decimal after the first digit.
1.67
Count the number of places you’d have to move that decimal to get back to the original number. In this case, that would be 10 places to the left. Therefore, you would multiply by a negative power of 10.
1.67 x 10-10 meters.
Let’s practice!
EXAMPLE 1
Write 180000000 in scientific notation.
First, write down the significant figures.
18
Next, put a decimal after the first digit.
1.8
Count the number of places you’d have to move that decimal to get back to the original number. In this case, that would be 8 places to the right.
1.8 x 108
EXAMPLE 2
Now try to write 0.0002200 in scientific notation.
Write down the significant figures.
2200
Put a decimal after the first digit.
2.200
Count the number of places you’d have to move that decimal to get back to the original number. In this case, that would be 4 places to the left.
2.200 x 10-4